See Additional Content Below
beamqlaser@gmail.com | info@beamq.com Chat box at bottom
Featured Products

15W Blue Laser Torch Pointers Flashlight Highest Real 15,000mW Output Power
$498.00 $490.00Save: 2% off

Portable Laser Dazzler Law Enforcement and Self Defense High Power Lasers for Sale
$490.00 $490.00Save: 0% off

465nm Sky Blue Laser Pointers Torch Output Power 3W 3000mW Water Proof Burning
$599.00 $199.00Save: 67% off
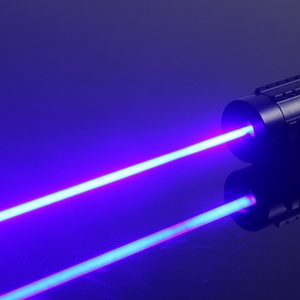
Real Output Power 5W 5000mW Blue Laser Torch Adjustable Focus Kaleidoscope
$259.00 $199.00Save: 23% off

Most Powerful 300-500mW Green Laser Rifle Sight Long Range Laser for AR15
$159.00 $129.00Save: 19% off
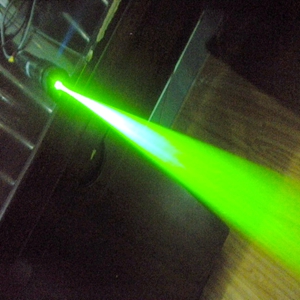
High Power Green Laser Dazzler Non-lethal Dazzler Weapon Eye Safe 532nm Green Beam
$560.00 $369.00Save: 34% off
Monthly Specials For April

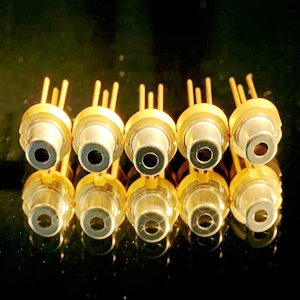
650nm 658nm Red Laser Diode LD 5.6mm ST TO56 for MOOG CT Laser Box HLM0610
$59.00 $39.00Save: 34% off











.jpg)